Also, if there is more than one exponential term in the function, the graph may look differentThe following are a couple of examples, just to show you how they work Graph y = 3×2 –x2 Because the power is a negative quadratic, the power is always negative (or zero) Then this graph should generally be pretty close to the x axisChapter 5 Functions Learners must be able to determine the equation of a function from a given graph Discuss and explain the characteristics of functions domain, range, intercepts with the axes, maximum and minimum values, symmetry, etcGraph of y = ax 2 bx c, where a and the discriminant b 2 − 4ac are positive, with Roots and yintercept in red;

Quadratic Function
Y=ax^2+c graph
Y=ax^2+c graph-Try varying the values of a and k and examine what effects this has on the graphThe graph of the equation y = ax2 bx c is a parabola congruent to the graph of y = ax2 Recall that a quadratic function is any function f whose equation can be put in the form f(x) = ax2 bx c, where a ≠ 0 Thus, the graph of every quadratic function is a parabola, with y–intercept f(0) = c



Graphing Y Ax 2 C
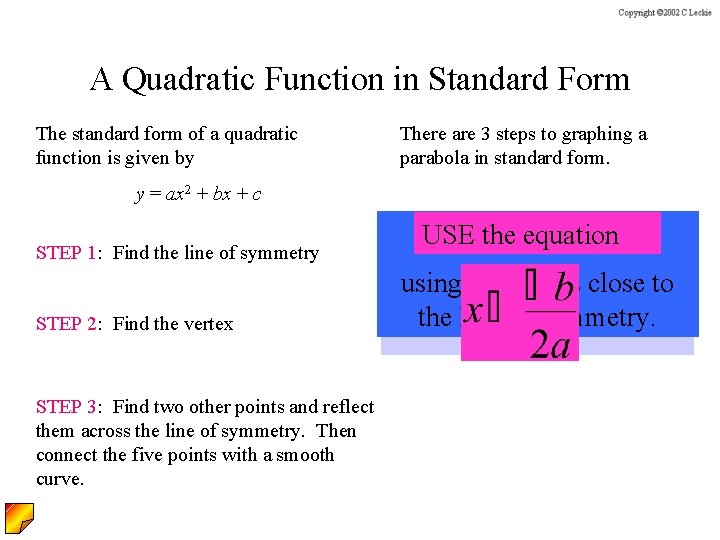
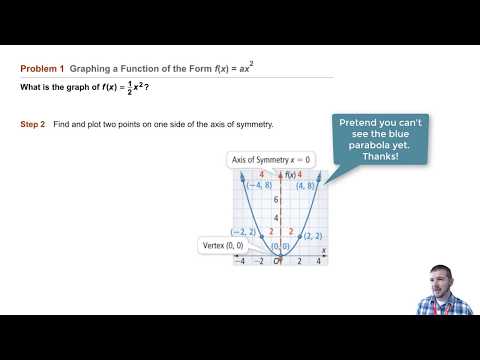
A quadratic function's graph is a parabola The graph of a quadratic function is a parabola The parabola can either be in "legs up" or "legs down" orientation We know that a quadratic equation will be in the form y = ax 2 bx c Our job is to find the values of a, b and c after first observing the graph Then you may graph 16 Problem 2 Formula y = ax2 bx c y = x2 4x 8 First we will find the vertex's xcoordinate using –b/2a –b/2a = 4/2 (1) = 4/2 = 2 Since 2 is our xcoordinate we will now endeavor to find our ycoordinate y = (2)2 4 (2) 8y=4–y = 4, so our vertex is at (2, 4) 17The graph of y=ax^2bxc is a parabola that opens up and has a vertex at (0,5) What is the solutio For problems 29 31 the graph of a quadratic function y=ax^2 bx c is shown
Vertex and axis of symmetry in blue; – Because the graph of the function y = ax is a straight line passing through the origin, we only need to define one more point A (usually for x=1;Here is the graph of y = a ( x − h) 2 k Use the sliders to change the values of a, h, and k so that you are looking at the graph of y = 2 ( x − 1) 2 2 Fill in the following table (The equation for the graph is written below the grid) Equation
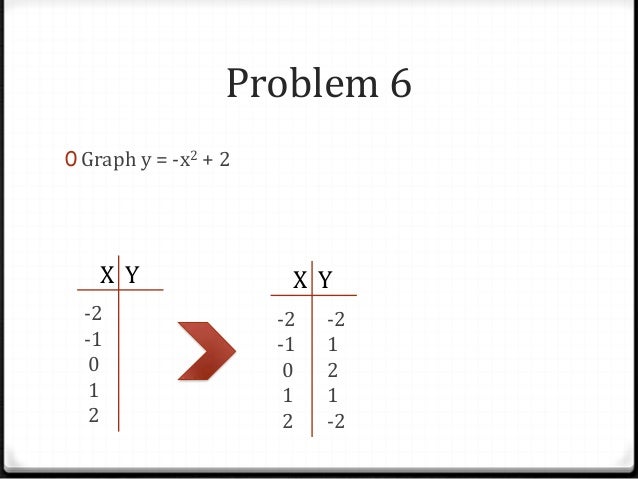
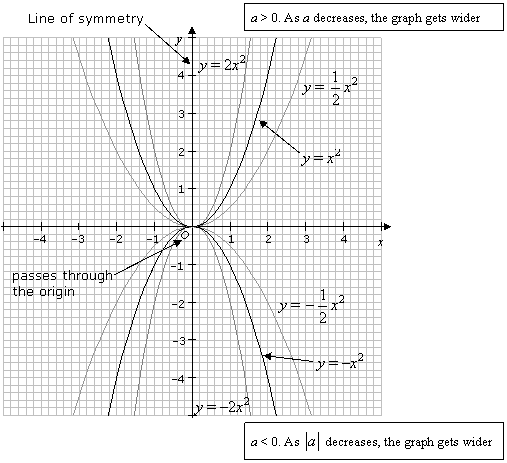
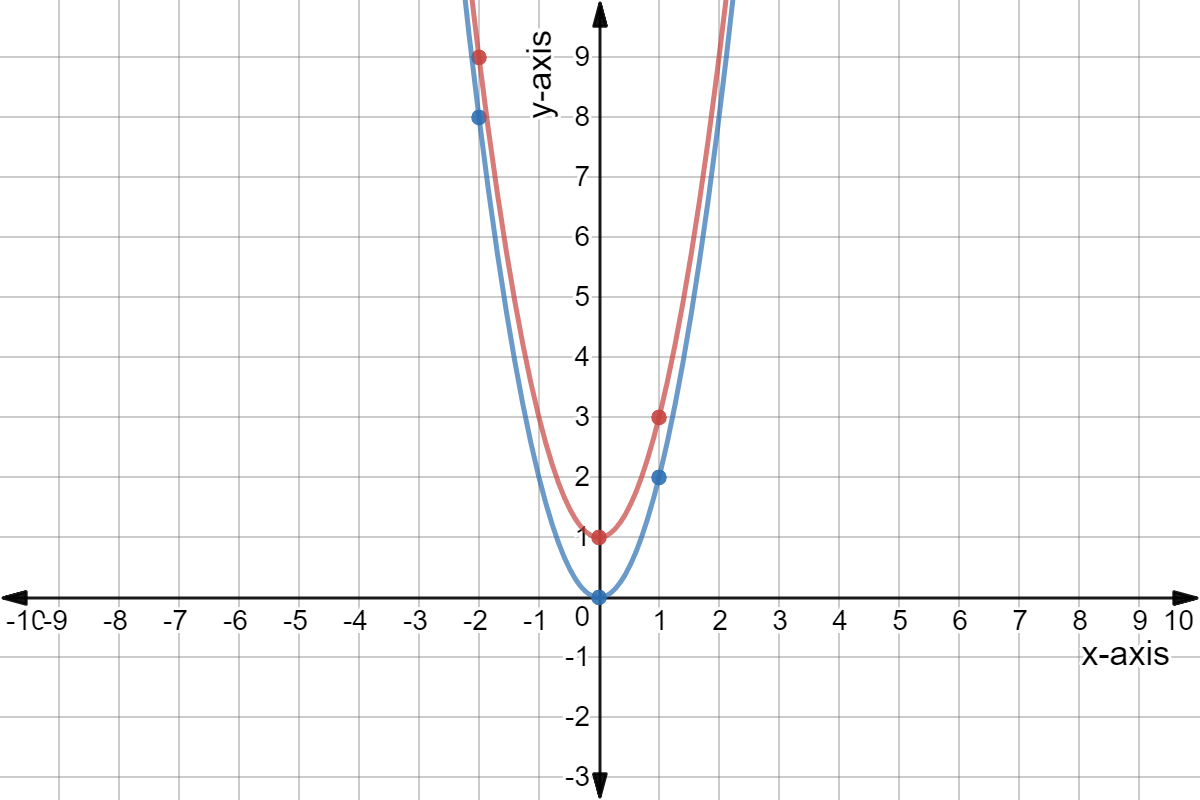
Choose a set of values of y Calculate corresponding x values and plot Draw a smooth curve through the plotted pointsGraphing y = ax^2 c 1 Problems0 Problem 1 Graph y = x Problem 2 Graph y = 2x Problem 3 Graph y = ½x Problem 4 Graph y = x Problem 5 Graph y = x2 40 Problem 6 Graph y = x2 Problem 7 Graph y = 2x2 4 2 Problem 10 Graph y = x2 3 Problem 10 Graph y = x2The first thing we need to do is to remember the x and ytableThe graph of a parabola should be roughly Ushaped in general How to Graph a Parabola of the Form {eq}y=ax^2c {/eq} Vocabulary Parabola This is the shape of the graph




Sketching Graphs Of The Form Y Ax2 Q Functions I



Quadratic Graph Example Y Ax Expii
Graph of y = ax2 bx c, where a and the discriminant b2 − 4ac are positive, with Roots and y intercept in red Vertex and axis of symmetry in blue Focus and directrix in pink Use the standard form y = ax^2bxc and the 3 points to write 3 equations with, a, b, and c as the variables and then solve for the variables Because the question specifies a function, we must discard the form that is not a function x = ay^2byc and use only the form y = ax^2bxc" 1" Using the point (0,3), we substitute 0 for x and 3 for y into equation 1 and theAn Exploration of the Graph of y=ax^2 Arguably, y = x^2 is the simplest of quadratic functions In this exploration, we will examine how making changes to the equation affects the graph of the function We will begin by adding a coefficient to x^2 The movie clip below animates the graph of y = nx^2 as n changes between 10 and 10



10 1 Quadratic Graphs Ppt Download



Pplato Basic Mathematics Quadratic Functions And Their Graphs
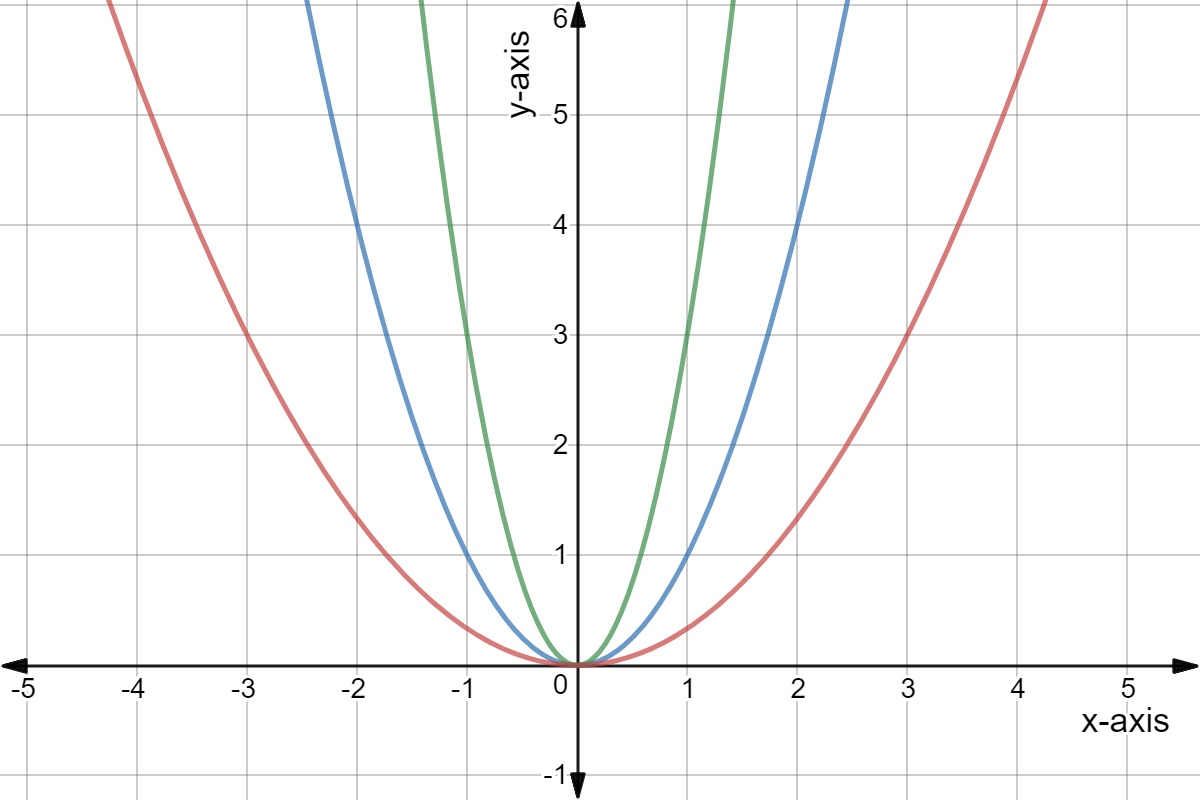
Graph of y=ax^2k Graph of Try varying the values of k and a and see how it affects the graph of this quadratic function Write down what you see as you change each value of 'a' and 'k' Explain why? Answer The solution set is ∅ Stepbystep explanation The expression y = ax^2 bx c is a quadratic equation The vertex is located at (2, 5) and the graph opens up, this means that it never intercepts the xaxis422 Chapter 8 Graphing Quadratic Functions Graphing y = ax2 When a < 0 Graph h(x) = − 1— 3 x2Compare the graph to the graph of f (x) = x2 SOLUTION Step 1 Make a table of values x −6 −30 3 6 h(x) −12 −30−3 −12 Step 2 Plot the ordered pairs Step 3 Draw a smooth curve through the points The graphs have the same vertex, (0, 0), and the same axis of symmetry, x = 0, but



Graphing Quadratic Functions Y Ax 2 Bx C




Act Math Practice Question 1184 Answer And Explanation Crackact Com
The graph of y=ax^2bxx is given below, where a,b , and c are integers Find abc I assume that is meant to be The graph of y=ax^2bx c is given below, where a,b , and c are integers Find abcA worksheet on the graph of the parabola y=ax^2 The exercises require to match a set of graphs (of parabolas) to their equations and find the equation of a parabola given its graph and a point on it Solutions are included From https//placeformathBelow you can see the graph of $y=x^26x$ The axis of symmetry of this parabola is the line $$x = {b}/{2a} = {(6)}/{2(1)} = 6/2 = 3$$ We want to find the vertex of this parabola The vertex is on the axis of symmetry, so its $x$coordinate is 3 The vertex is also a point on the parabola, so it satisfies the equation for the parabola




Find The Quadratic Function Y Ax2 Bx C Whose Chegg Com



1
Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeThis is the graph of the equation y = 0x 3 0x 2 0x12 This simplifies to y = 12 and so the function has the value 12 for all values of x It is therefore a straight horizontal line through 12 on the y axis Play with different values of d and observe the result Linear equations (y = cx) Click 'zero' on all four sliders;The graph of a quadratic equation in two variables (y = ax 2 bx c) is called a parabolaThe following graphs are two typical parabolas their xintercepts are marked by red dots, their yintercepts are marked by a pink dot, and the vertex of each parabola is marked by a green dot



Quadratic Graph Example Y Ax Expii
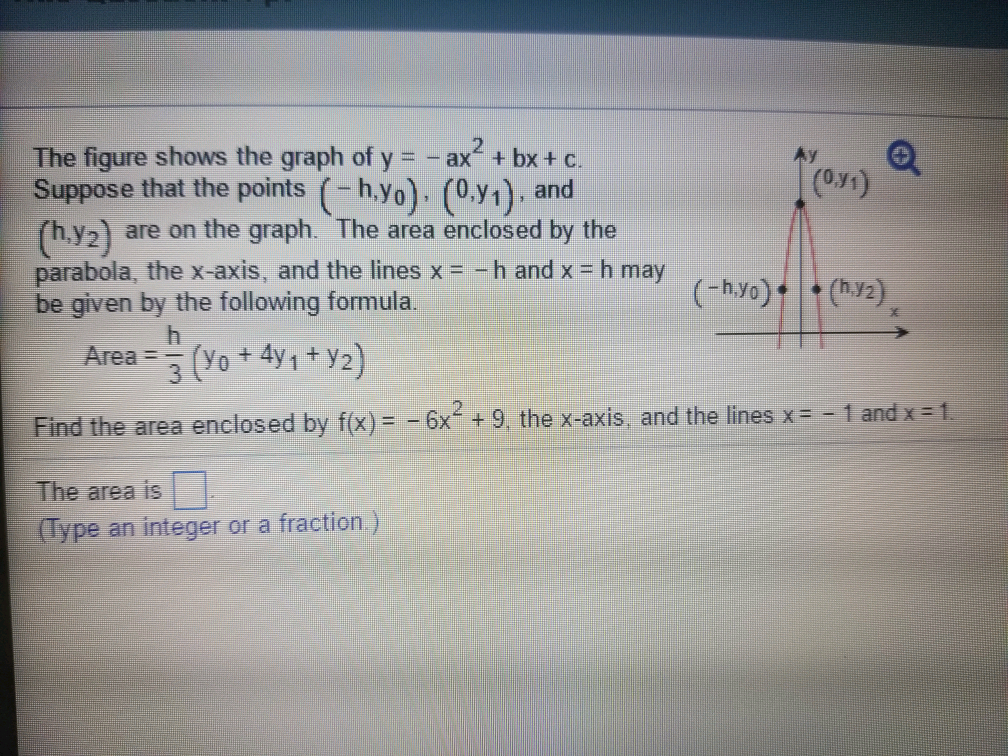



The Figure Shows The Graph Of Y Ax 2 Bx C Chegg Com
Find stepbystep Algebra solutions and your answer to the following textbook question Find a and b if the graph of $$ y = ax^2 bx^3 $$ is symmetric with respect to the origin (There are many correct answers)A quadratic function in the form f (x) = ax2 bxx f ( x) = a x 2 b x x is in standard form Regardless of the format, the graph of a quadratic function is a parabola The graph of y=x2−4x3 y = x 2 − 4 x 3 The graph of any quadratic equation is always a parabola The axis of symmetry is the vertical line passing through the middle of the parabola \(y=ax^2bxc\) parabola The graph of a quadratic equation in two variables is a parabola quadratic equation in two variables A quadratic equation in two variables, where a, b, and c are real numbers and \(a \ge 0\) is an equation of the form \(y=ax^2bxc



Quadratic Graph Example Y Ax C Expii
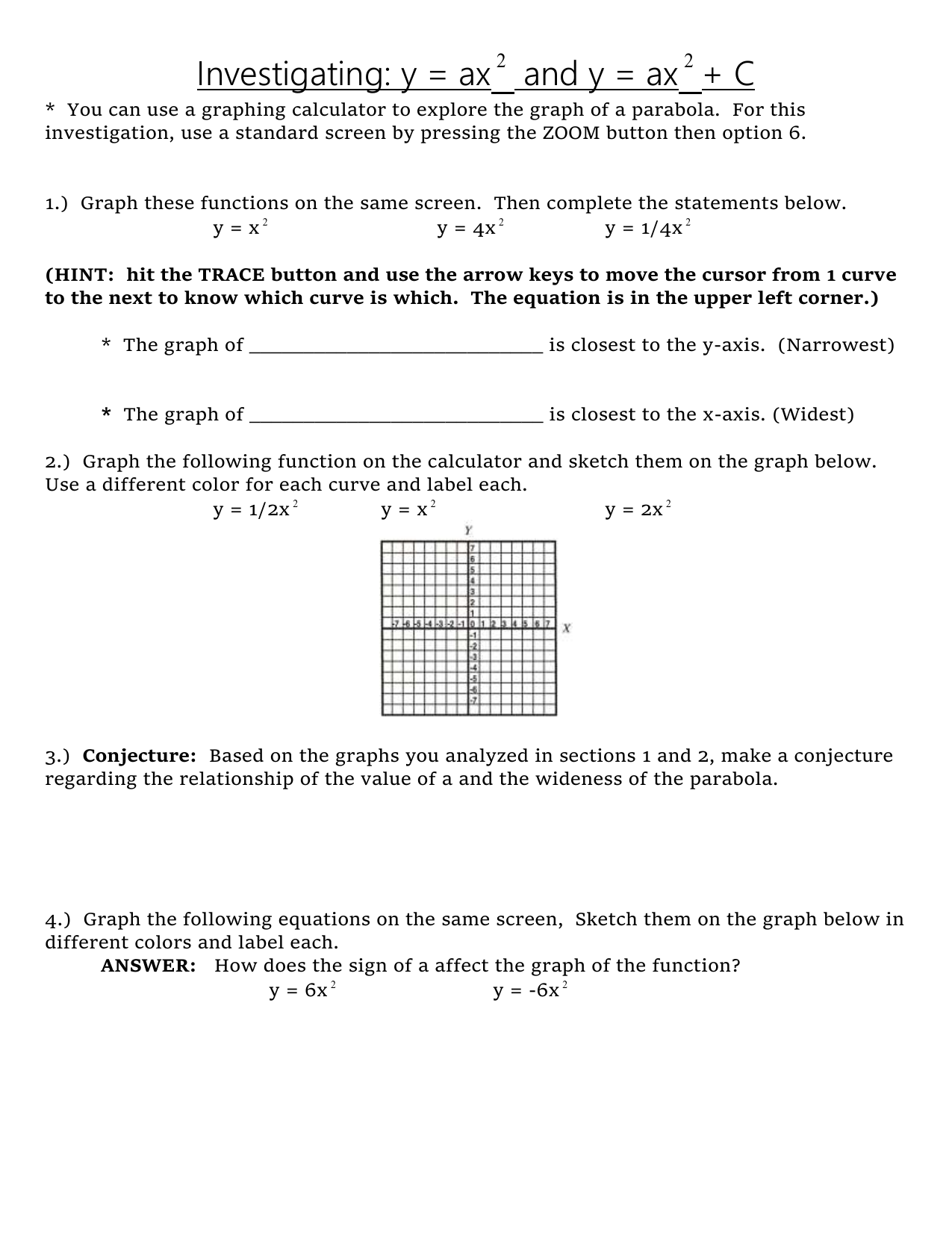



Investigating Y Ax And Y Ax C 2
Explorations of the graph y = ax 2 bx c In this exercise, we will be exploring parabolic graphs of the form y = ax 2 bx c, where a, b, and c are rational numbers In particular, we will examine what happens to the graph as we fix 2 of the values for a, b, or c, and vary the third We have split it up into three parts varying a only varying b onlyGraph y=x^2 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for The focus of a parabola can be found by adding to the ycoordinate if the parabola opens1 2 HW #92 Graphing y = ax3 Graph the following cubic equations Follow the given steps Steps 1 Make an (x, y) t – chart 2 Choose five values to substitute for x 3 Plug the values for x into the given equation, determining the y values 4 Plot the five ordered pairs 5 Connect the ordered pairs with a smooth curve



Graphing Quadratic Functions Examples Solutions Videos



Assignment 2 Investigating The Relationship Between The Two Standard Forms Of The Graph Of A Parabola
The graph of y = ax 2 bx c is shown below determine the solution set of 0 The graph of y = ax 2 bx c is shown below determine the solution set of 0 = ax 2 bx c Categories Uncategorized The graph of the equation y= ax^2, where a is > O, is shown below, If a is multiplied by 1/2, the graph of the new equation is a A widet and opens downwardAlgebra Quadratic Equations and Functions Quadratic Functions and Their Graphs 1 Answer Douglas K




Graphing Y Ax 2 C



Quadratic Graph Example Y Ax Expii
A worksheet on the graph of the parabola y=ax^2 The exercises require to match a set of graphs (of parabolas) to their equations and find the equation of a parabola given its graph and a point on it Solutions are included From https//placeformath Here is the graph of y = (x − 1) 2 Example 5 y = (x 2) 2 With similar reasoning to the last example, I know that my curve is going to be completely above the xaxis, except at x = −2 The "plus 2" in brackets has the effect of moving our parabola 2 units to the left Rotating the Parabola The original question from Anuja asked how toGiven equation y^2 = Note that the origin (0,0) is a point on the graph Graph is symmetric about x axis (Both y and y are mapped to the same x value) To draw the graph, Choose a value for a;



Example 1 Graph A Function Of The Form Y Ax 2 Graph Y 2x 2 Compare The Graph With The Graph Of Y X 2 Solution Step 1 Make A Table Of Values For Ppt Download



Ppt Recall The Graph Of A Quadratic Function Y Ax 2 Bx C Is A Parabola Powerpoint Presentation Id
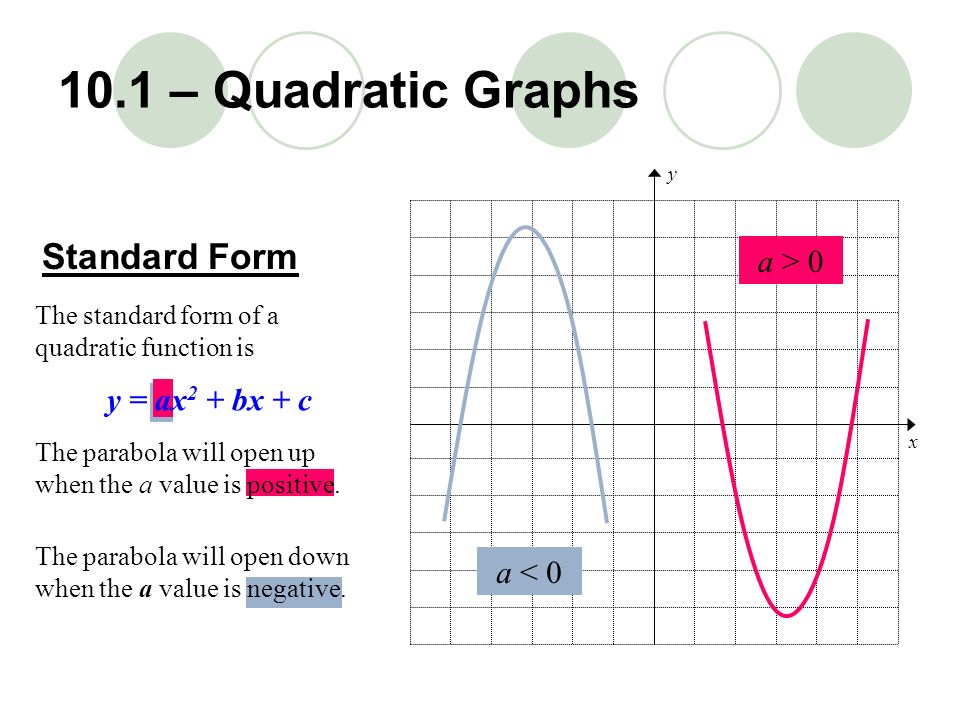
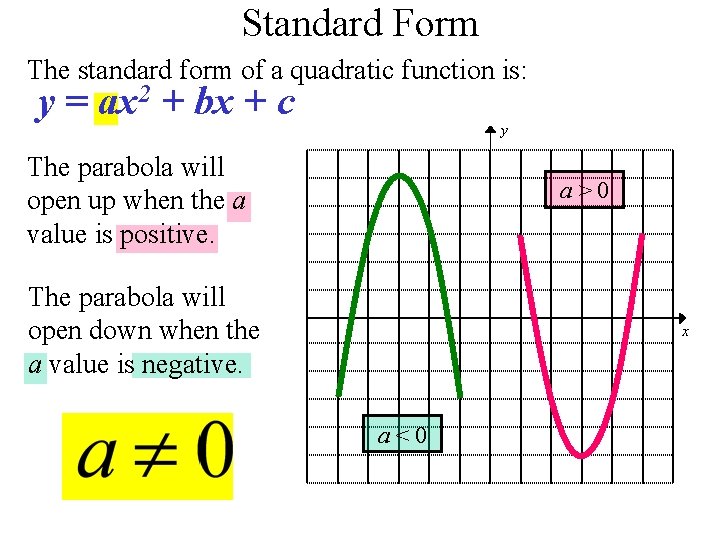
Algebra Graph y=ax^2 y = ax2 y = a x 2 Find the standard form of the hyperbola Tap for more steps Subtract a x 2 a x 2 from both sides of the equation y − a x 2 = 0 y a x 2 = 0 Divide each term by 0 0 to make the right side equal to one y 0 − a x 2 0 = 0 0 y 0 a x 2 0 = 0 0The general form of a quadratic is "y = ax 2 bx c"For graphing, the leading coefficient "a" indicates how "fat" or how "skinny" the parabola will beFor a > 1 (such as a = 3 or a = –4), the parabola will be "skinny", because it grows more quickly (three times as fast or four times as fast, respectively, in the case of our sample valuesGraphs of quadratic functions All quadratic functions have the same type of curved graphs with a line of symmetry The graph of the quadratic function \(y = ax^2




Quadratic Function



We Know How To Graph The Quadratic Equation Y X2
The graph of y=x^22x1 is translated by the vector (2 3)The graph so obtained is reflected in the xaxis and finally it is stretched by a factor 2 parallel to yaxisFind the equation of the final graph is the form y=ax^2bxc, Calculus really quick check The graph of f ′(x) is continuous and decreasing with an xintercept at x = 2 The diagram below shows a sketch of part of the graph y = ax bx 3 The two points V and W each lie on the graph and have coordinates (1,10) and (213) W (2,13) V (1,10) Diagram is NOT to scale Find the values of the numbers a and b How do you find the quadratic function #y=ax^2 bx c# whose graph passes through the given points (1, 4), (1, 12), (3, 12)?
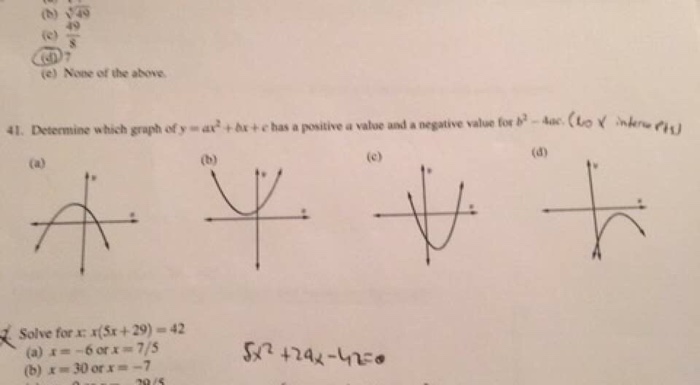



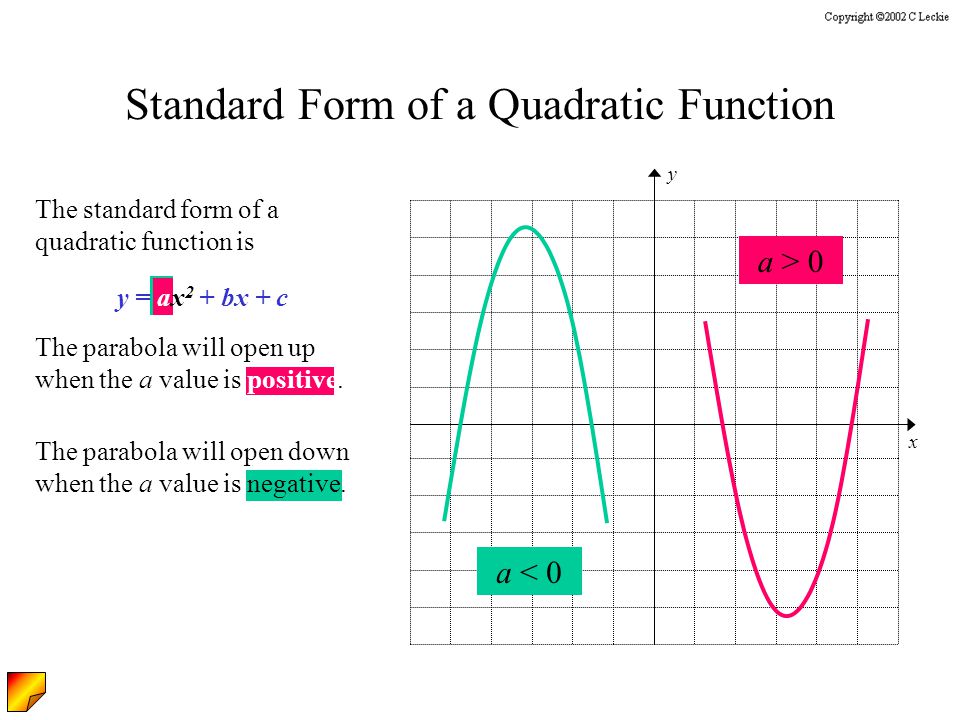
Determine Which Graph Of Y Ax 2 Bx C Has A Chegg Com



1
Likewise y= y'y_0 Solve for x' and y' and plug into y'=ax'2, get (yy_0)=a(xx_0)^2, now you are back in the original systemYour b =2ax_0, where x_0 is the xcoordinate of the vertexLet me know if ok $\endgroup$ – Peter Szilas Sep 27 '18 at 1756Move the c sliderFocus and directrix in pink;




The Graph Of Y Ax 2 Bx C Is Shown Below Determine The Solution Set Of 0 Ax 2 Bx C Brainly Com



Lesson 10 2 Quadratic Functions And Their Graphs Y Ax 2 Bx C Ppt Download
Visualisation of the complex roots of y = ax 2 bx c the parabola is rotated 180° about its vertex (orange)I The graph of y = – x 2 ax b cuts the xaxis at two points II (a – 1) 2 4 b > 4 c III b < c A I only B I and II only C I and III only D II and III only 23 In ABC, AB = 8, BC = 9 and AC = 6 Find sin AA free graphing calculator graph function, examine intersection points, find maximum and minimum and much more This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Weekly Subscription $2



Algebra 1 Activity Sheet On Graphing Y Ax 2 C By Math Through Discovery Llc
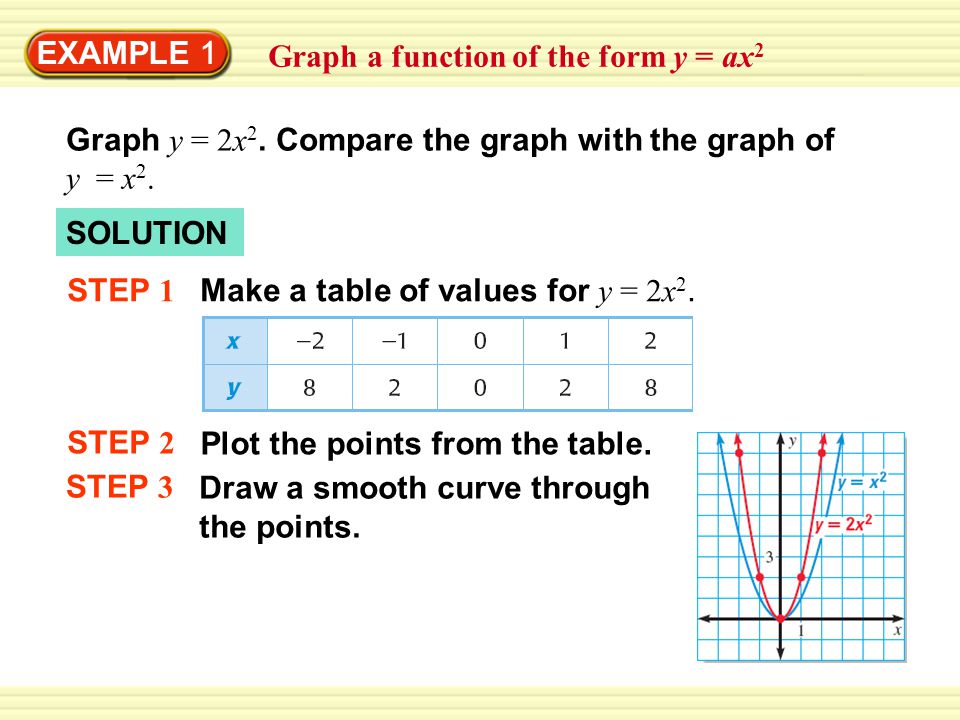



Example 1 Graph A Function Of The Form Y Ax 2 Graph Y 2x 2 Compare The Graph With The Graph Of Y X 2 Solution Step 1 Make A Table Of Values For Ppt Download
The graph of the quadratic trinomial y = a x 2 b x c has its vertex at (4, − 5) and two xintercepts, one positive and one negative Which of the following holds good?This question has multiple correct optionsY=a) different from the origin O Draw the line OA we get the graph of the function y = ax 2 Illustrated exercise



What Are The Coefficients A B And C Of The Parabola Y Ax 2 Bx C That Passes Through The Point 3 13 And Tangent To The Line 8x Y 15 At 2 1 Quora



Solution A Quadratic Graph Has Maximum Point 2 5 And Passes Through Point 0 3 Find The Equation For The Graph In The Form Y Ax 2 Bx C
If the graphs of y = – x 2 ax b and y = x c do not intersect with each other, which of the following must be true?
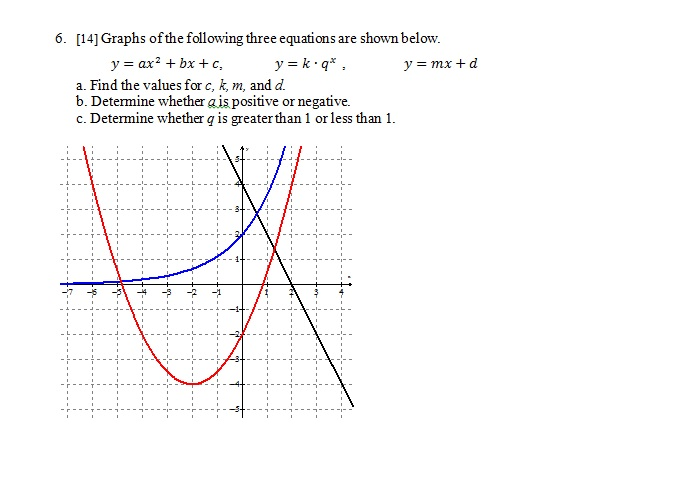



Graphs Of The Following Three Equations Are Shown Chegg Com



Assignment 2 By Shridevi Kotta This Write Up Explores Equation Of Parabola Y Ax2 Bx C We Observe That With B C 0 A Determines How The Parabola Is Oriented And The Curve Rises Fast Or Slow In Other Words How Narrow Or How Wide The Parabola Is At



To Investigate The Effect Of The Constants A B And C On The Graph Y Ax2 Bx C Gcse Maths Marked By Teachers Com




Power Function Graph Y Ax Geogebra



Solution Draw A Sketch Graph Of Y Ax 2 Bx C If A Lt 0 B Lt 0 C Lt 0 Discriminant 0



Exploring Parabolas Y Ax 2 Bx C
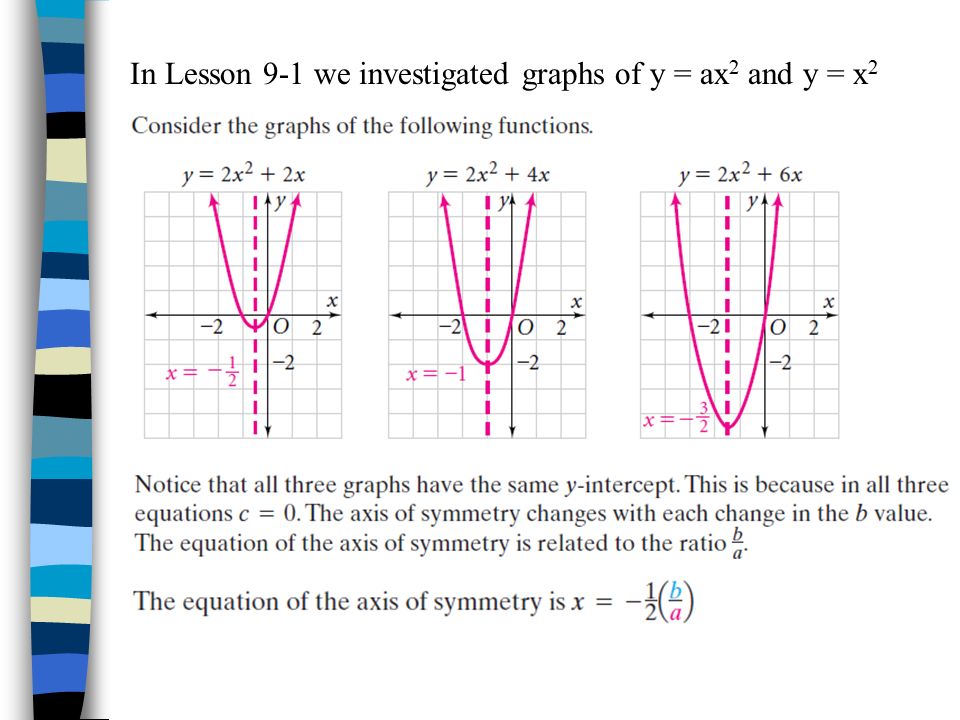



Lesson 9 2 Graphing Y Ax Bx C Objective To Graph Equations Of The Form F X Ax Bx C And Interpret These Graphs Ppt Download
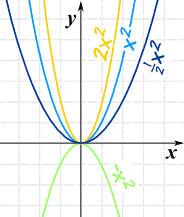



Graphing Quadratic Equations




Analyzing The Graph Of Y Ax 2 A 0 Geogebra
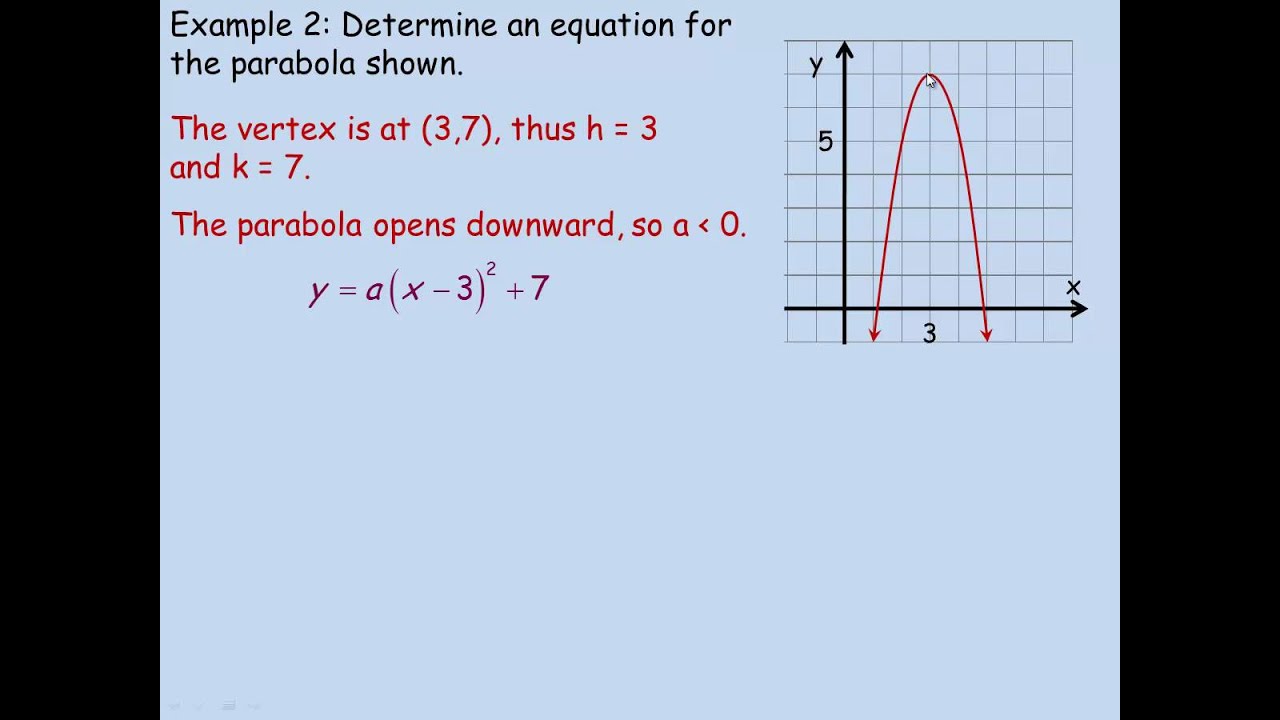



Graphing Y A X H K Youtube



Quadratic Graph Example Y Ax Expii



Quadratic Graph Example Y Ax C Expii



Graphing Y Ax 2 C




The Graph Of The Quadratic Function Y Ax 2 Bx C Is Given Find A B C Brainly Com



1



The Graph Of Y Ax 2 Bx C Has A Minimum At 5 3 And Passes Through 4 0 How Do I Find The Values Of A B And C Quora



Quadratic Function Y Ax 2 Bx C Quadratic
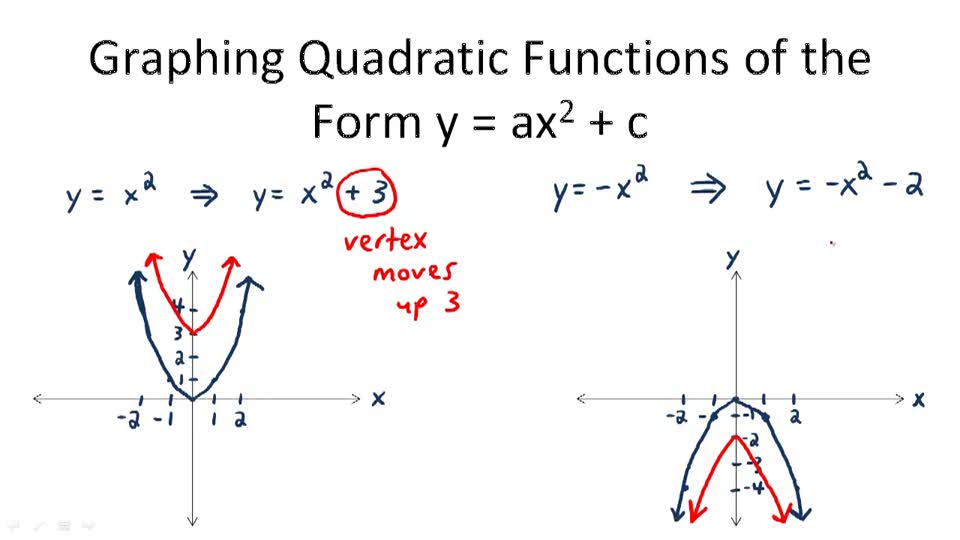



Graphing Quadratic Functions Of The Form Y Ax 2 C Overview Video Algebra Ck 12 Foundation



Graphing A Quadratic Function A Quadratic Function Has




Quadratic Function Wikipedia



Graphing Quadratic Functions Y 2 Ax Bx C
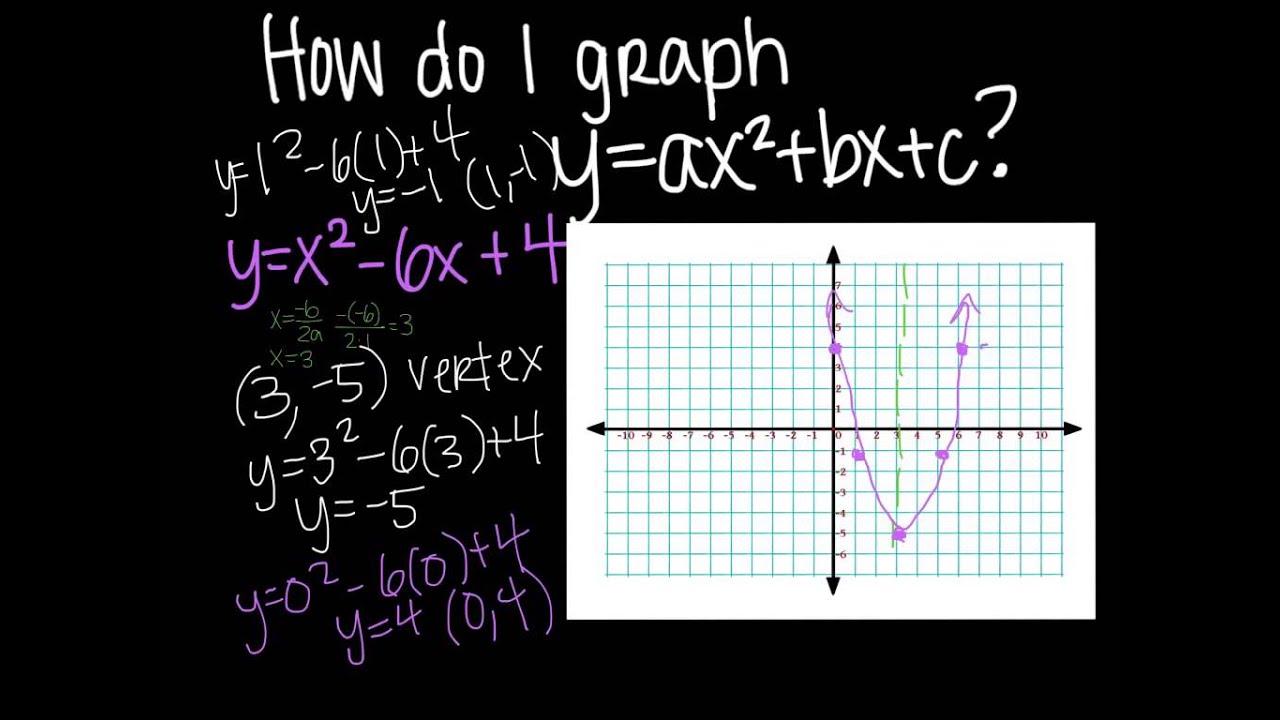



9 2 Graphing Y Ax2 Bx C Youtube
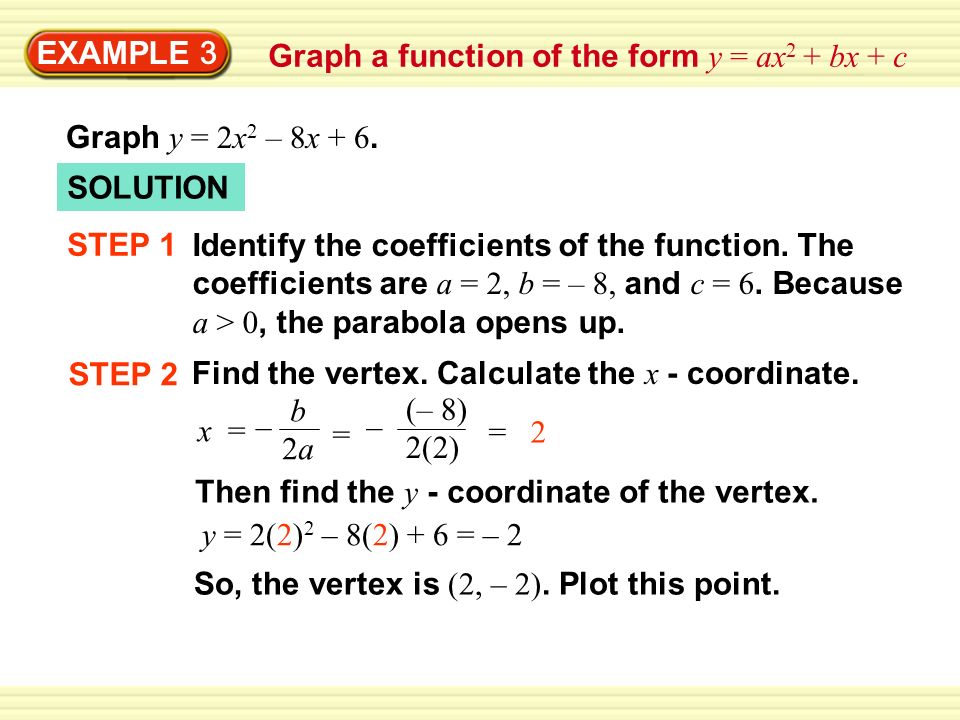



Example 3 Graph A Function Of The Form Y Ax 2 Bx C Graph Y 2x 2 8x 6 Solution Identify The Coefficients Of The Function The Coefficients Ppt Download
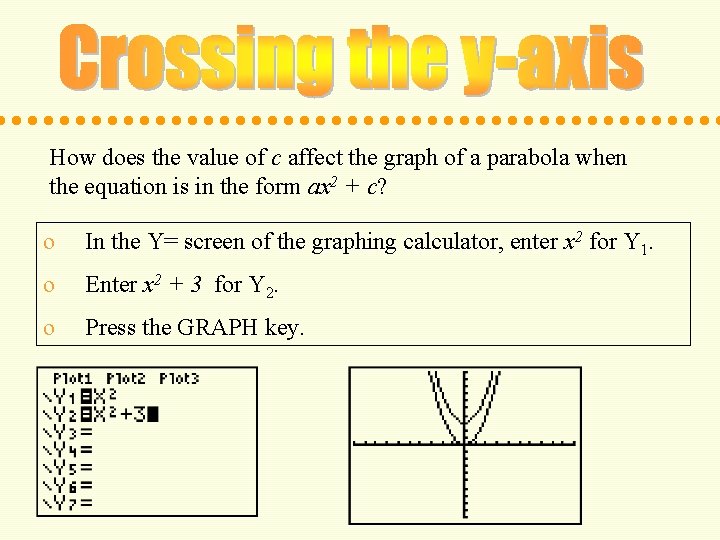



Quadratic Equation Equation In The Form Yax 2
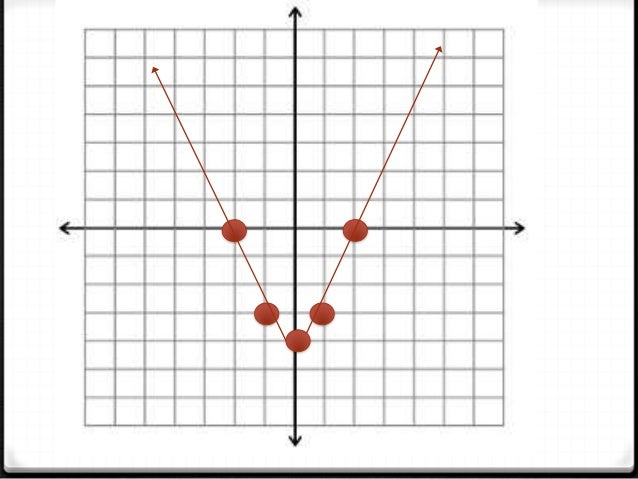



Graphing Y Ax 2 C




Graphing Y Ax 2 C



An Exploration Of The Graph Of Y Ax 2 Font
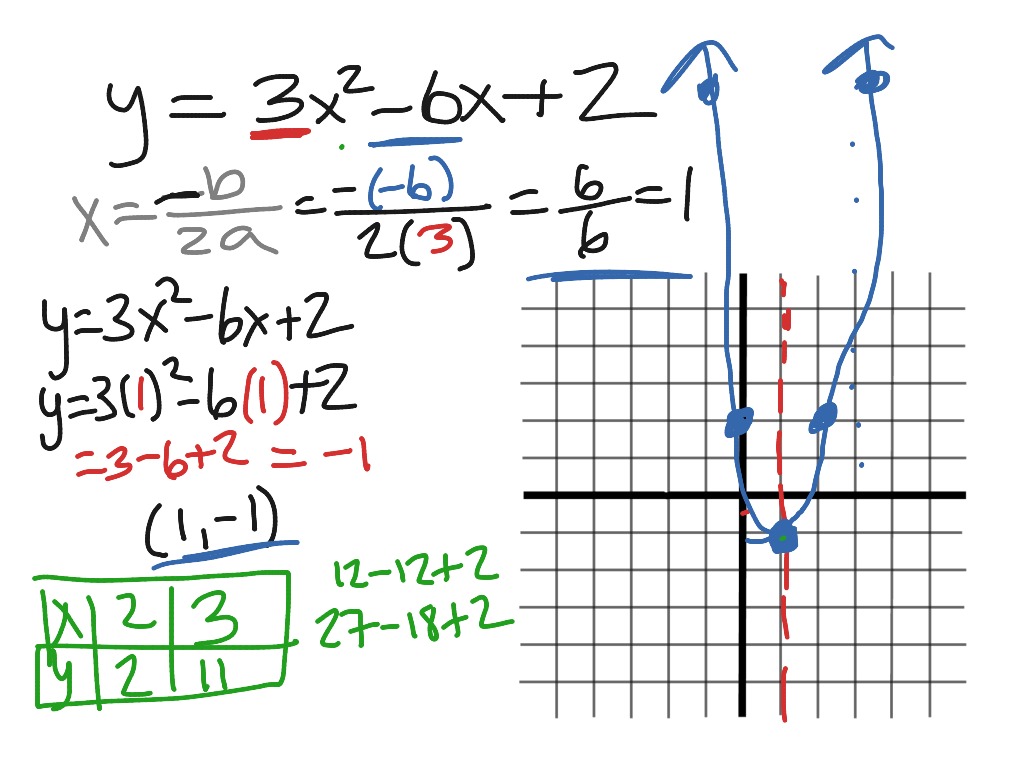



10 2 Graph Ax 2 Bx C Math Showme



Graphing Y Ax2 Bx C Youtube
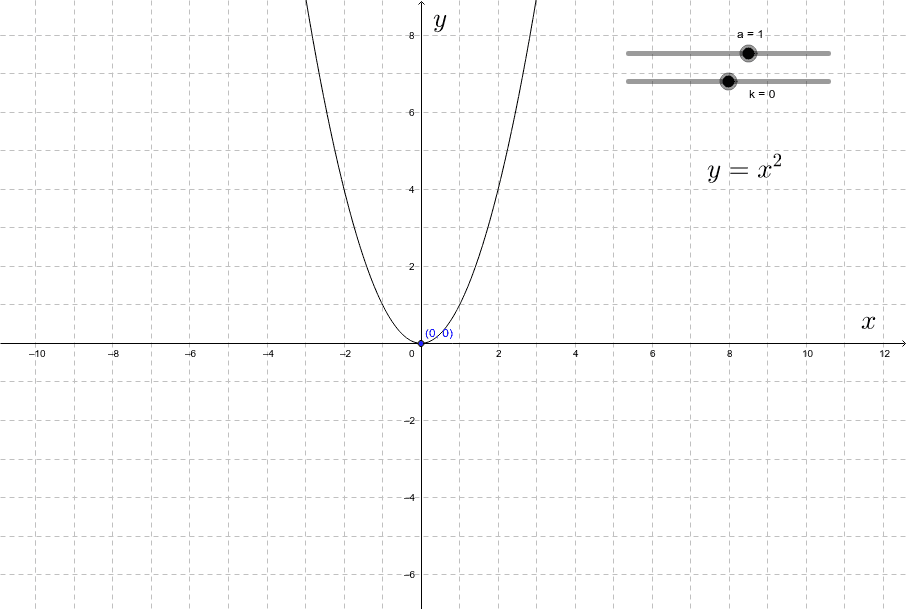



Graph Of Y Ax 2 K Geogebra



Graphing Quadratic Functions
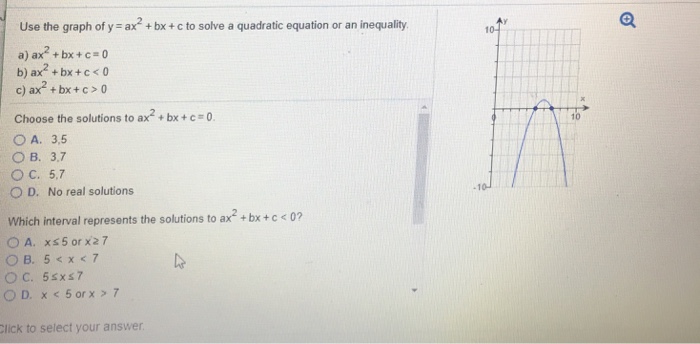



Use The Graph Of Y Ax 2 Bx C To Solve A Chegg Com
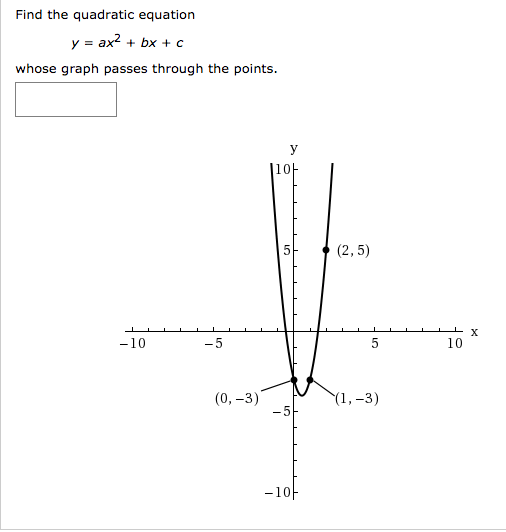



Find The Quadratic Equation Y Ax2 Bx C Whose Chegg Com




Number Of A Parabola Of The Form Y Ax 2 Bx C With A 0 Intersection S Of These Graph Of F X 1 X 2 4 Number Of A Possible Distinct Intersection S Of These Graph Is




The Graph Of The Polynomial Y Ax2 Bx C Is Shown In Fig 2 2 Write The Value Of B2 4ac Y Ax2 Bx C Own Classes




2 Graph Of Y Ax 2 Bx K Graph Of Y Mx K Download Scientific Diagram



Graphing Parabola Y Ax 2 Youtube



Graphing Parabolas In The Form Y Ax 2 Youtube



Graphing Y Ax 2 C Activity Builder By Desmos



The Given Figure Shows The Graph Of The Polynomial F X Ax 2 Bx C Then Ltbr Gt 1 Nbsp Youtube
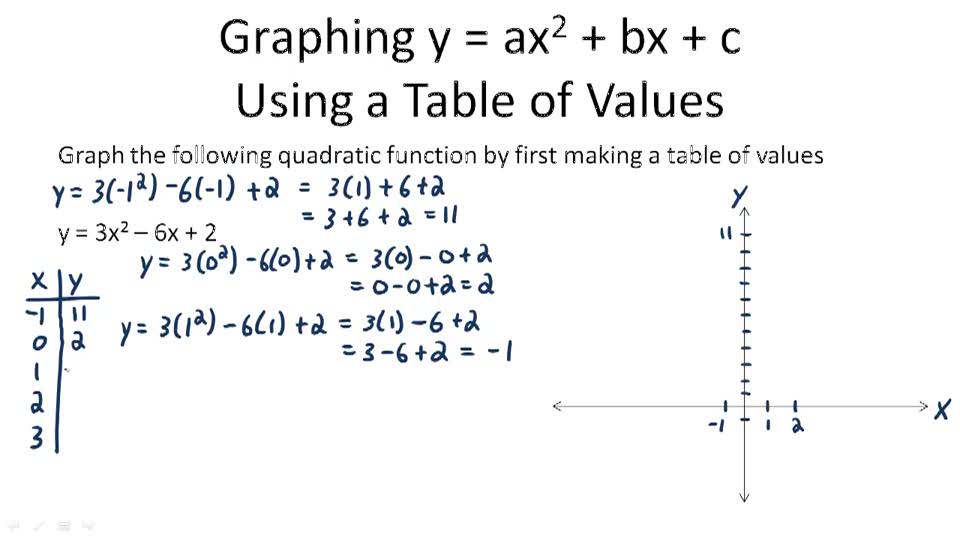



Graphing Quadratic Functions Of The Form Y Ax 2 Bx C Example 1 Video Algebra Ck 12 Foundation
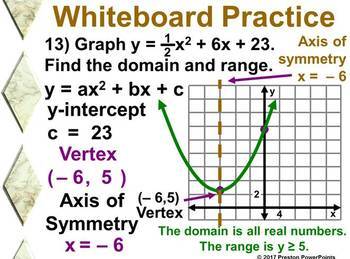



Alg 1 Graphing Y Ax2 Bx C In A Powerpoint Presentation Tpt
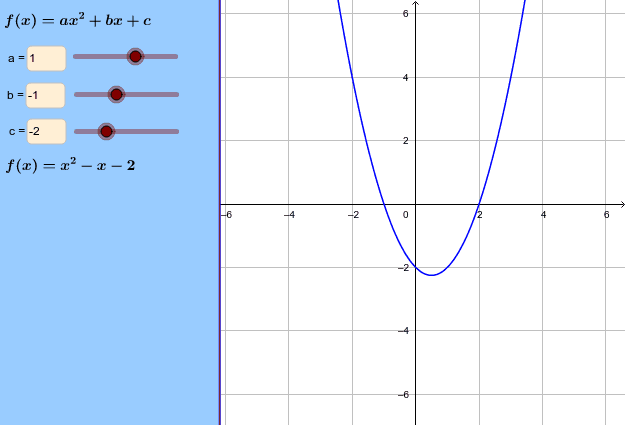



The Graph Of Y Ax 2 Bx C Geogebra




5 1 Quadratic Functions Functions Siyavula



Unique Quadratic Equation In The Form Y Ax 2 Bx C




If A 0 And B 2 4ac 0 Then The Graph Of Y Ax 2 Bx C



Quadratics
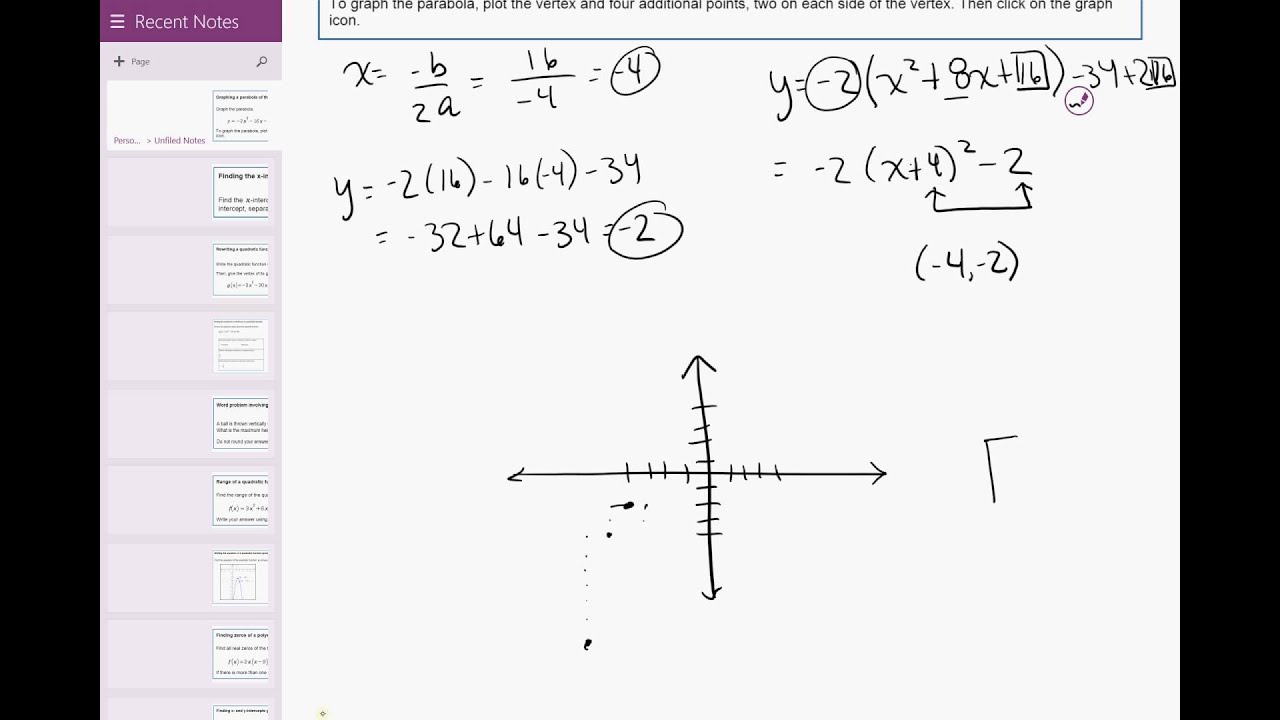



15 04 Graphing A Parabola Of The Form Y Ax 2 Bx C Integer Coefficients Youtube



How Does A Quadratic Graph In The Form Of Y Ax 2 Appear When A Is A Negative Number Quora



An Exploration Of The Graph Of Y Ax 2 Font



Examining The Parabola Assignment 2
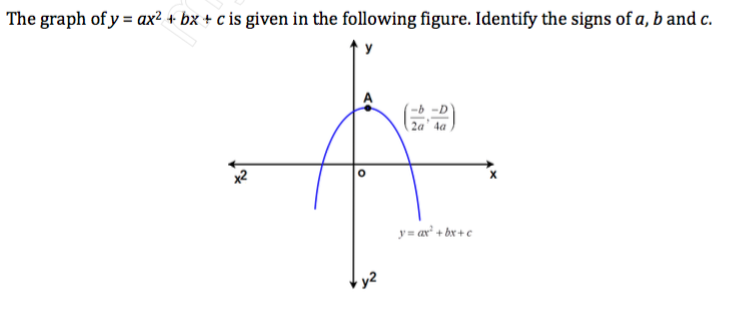



The Graph Of Y Ax 2 Bx C Is Given In The Chegg Com



Algebra 1 Activity Sheet Understanding How B Affects The Graph Of Y Ax 2 Bx C



1




Mbf3c 4 2 The Quadratic Relation Y Ax 2 K Youtube




Algebra1 Graphing Y Ax 2 Youtube



Pplato Basic Mathematics Quadratic Functions And Their Graphs




Quadratic Function



Pplato Basic Mathematics Quadratic Functions And Their Graphs



Quadratics




Snapshot Of A Sim Lesson Showing The Graph Of Y Ax 2 Download Scientific Diagram



Graphing Quadratics With Vertex And Axis Of Symmetry At The End Of The Period You Will Learn 1 To Compare Parabola By The Coefficient 2 To Find The Ppt Download



Quadratics




Quadratic Function Wikipedia
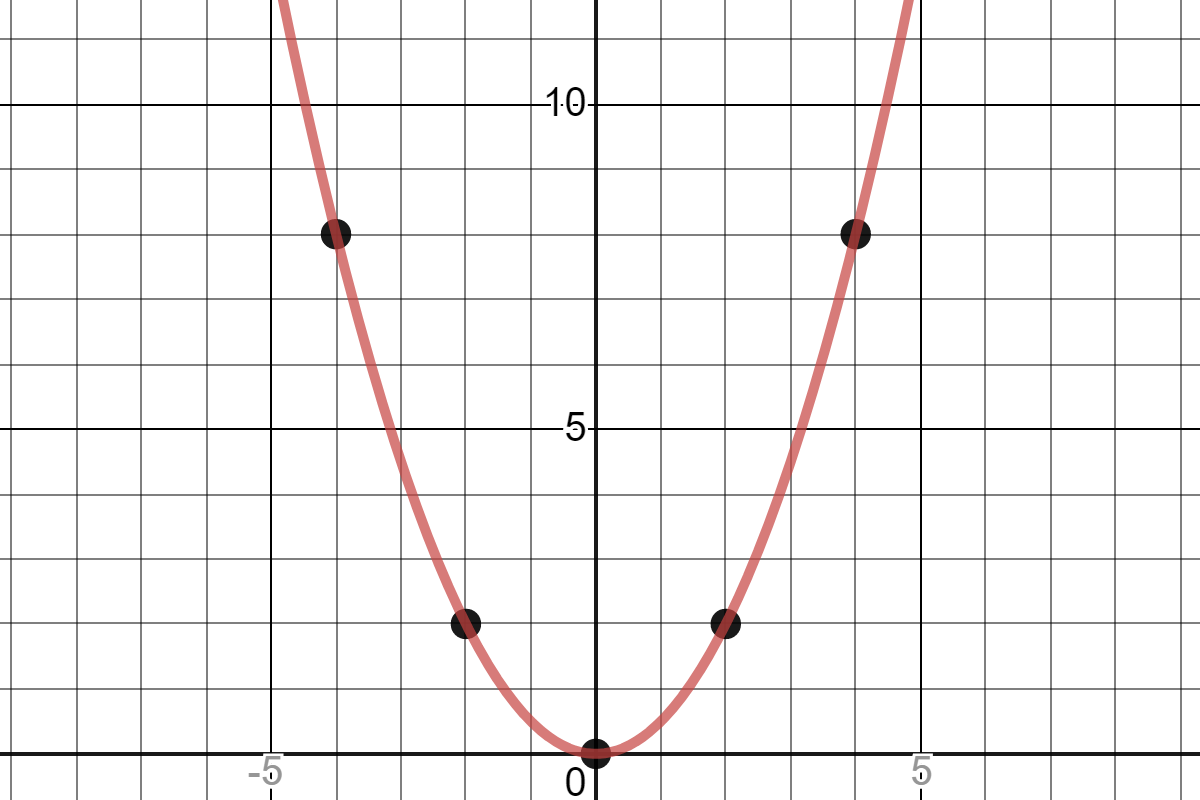



Quadratic Graph Example Y Ax Expii




This Is What The Problem Says Assuming All Parabolas Are Of The Form Y Ax 2 Bx C Drag And Drop The Brainly Com
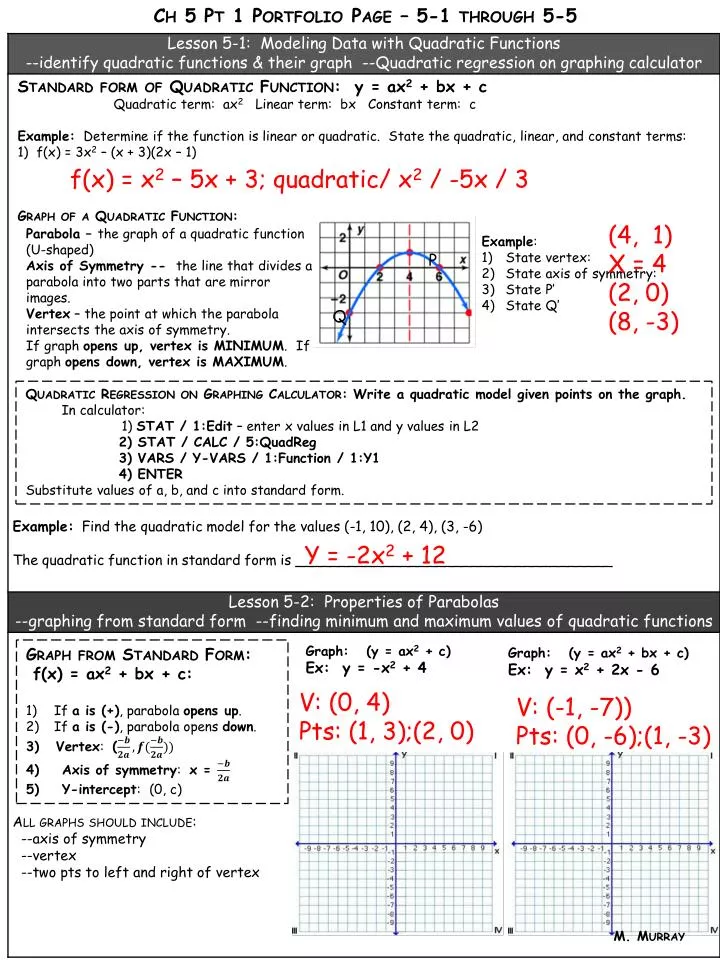



Ppt Standard Form Of Quadratic Function Y Ax 2 Bx C Powerpoint Presentation Id
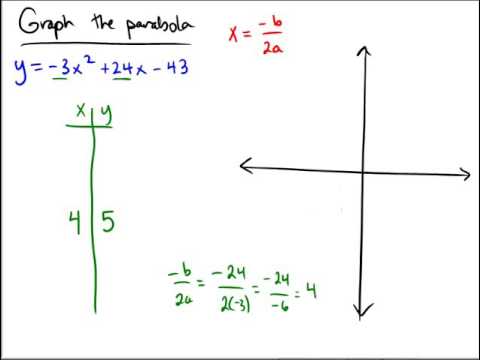



Graphing A Parabola Of The Form Y Ax 2 Bx C Integer Coefficients Youtube




Graphing A Parabola Of The Form Y Ax2 Bx C With Integer Coefficients Youtube




Use The Graph Of Y Ax2 Bx C To Solve A Chegg Com




If Graph Of Y Ax 2 Bx C Is As Shown Then Which Of The Following Is Correct Where D Equals B 2 4ac



Power Function Graph Y Ax 3 Geogebra
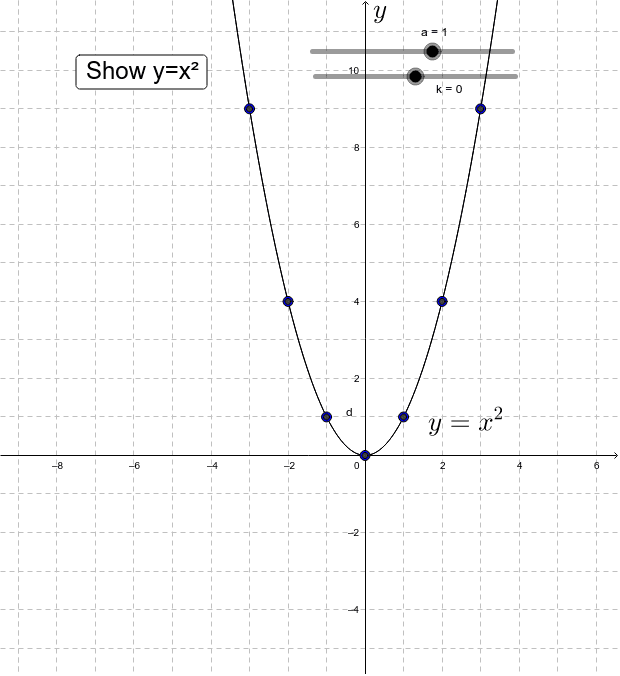



Graph Of Y Ax 2 K Geogebra



Assignment 2



We Know How To Graph The Quadratic Equation Y X2




Graphing Y Ax 2 Bx C



0 件のコメント:
コメントを投稿